Uncertainty quantification for PDEs on hypergraphs
Duration: 09/2023 – 08/2027
Funding scheme: Academy Research Fellow
Funder: Academy of Finland
Budget: 667,532 € + 286,086 €
My role: Principal investigator
Link: https://research.fi/en/results/funding/78093
Public description
3D printing is becoming ubiquitous in engineering and science. One of the main reasons for such success is its ability to create small structures not producible in any other known way. Typical examples include lightweight but strong materials (resembling, e.g., honeycombs) and artificial tissue. Such materials need specific properties, while production is subject to uncertainties in the printing process. This project grows out of the need for mathematical algorithms to find optimal structures that retain their outstanding properties even in the presence of small errors. Robustness is vital for lightweight materials used to build lighter cars, planes, and rockets that save fuel. Similarly, 3D-printed artificial tissue has to mimic the real human tissue of fire victims to a high degree.
Compared to the most efficient existing approaches, the methodology of this project reduces the cost of an optimization-based product design cycle by orders of magnitude.
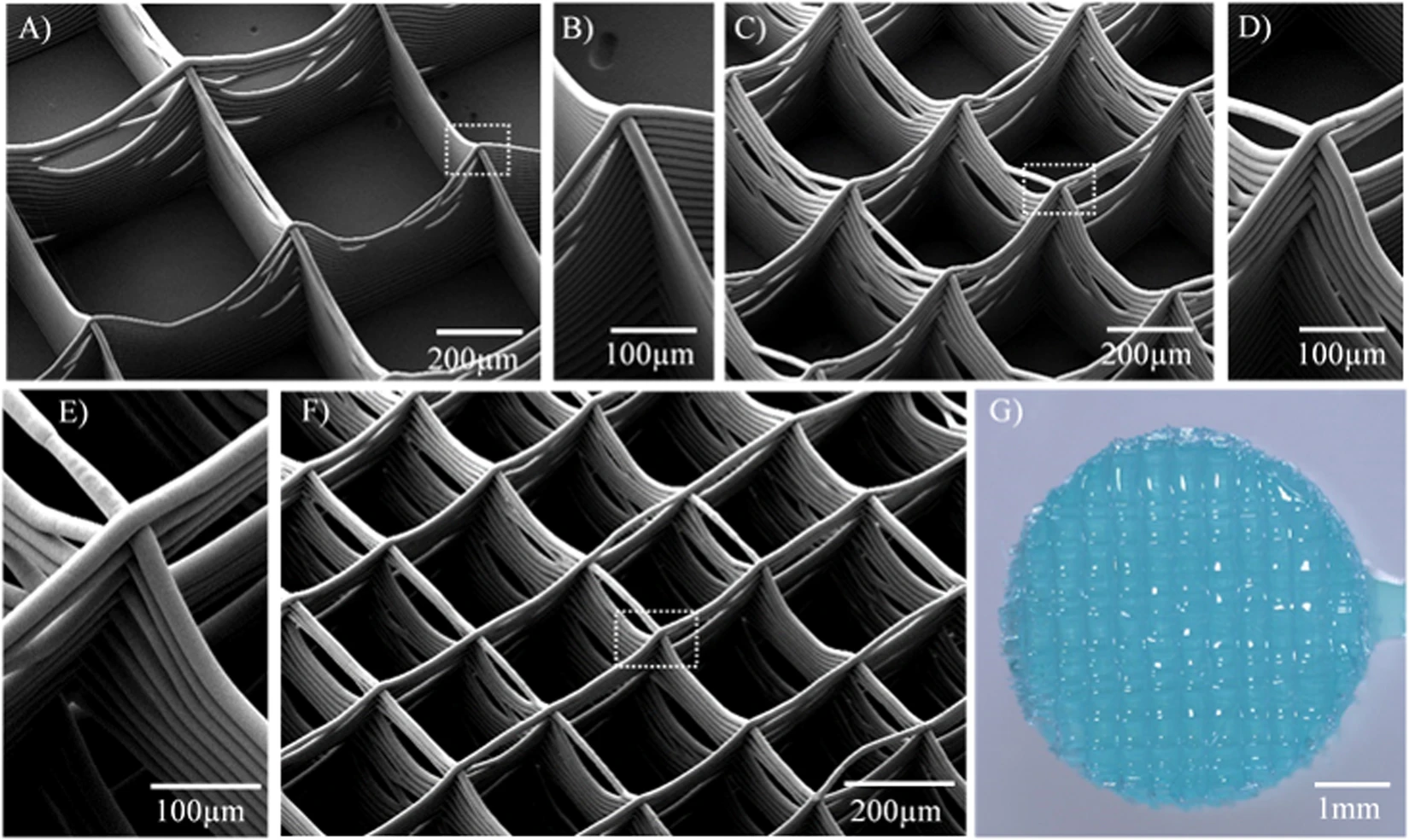
The picture shows micro-fiber scaffolds that are one artificial tissue component. It is taken from Mechanical behavior of a soft hydrogel reinforced with three-dimensional printed microfibre scaffolds by Miguel Castilho et al., licensed under CC BY 4.0.
Scientific abstract
Modeling such 3D printed objects is not feasible using traditional approaches: On the one hand, they cannot be interpreted as graphs, for which low-cost simulations could be run many times to find an optimal design. On the other hand, the cost of statistics-based optimization using full three-dimensional models is prohibitively high.
This project tackles this issue and gives rise to a new area of mathematics at the interface of applied mathematics and statistics. It uses networks of surfaces, so-called ‘hypergraphs,’ to describe the geometry and topology of 3D printed objects and poses partial differential equations that describe the physical properties of these hypergraphs. We discretize the partial differential equations with hybrid discontinuous Galerkin methods and use Monte Carlo-based statistics to quantify uncertainty. Moreover, we shape-optimize the hypergraph to achieve specific material properties.
Keywords
complex domains, hybrid discontinuous Galerkin, hypergraphs, multilevel Monte Carlo, quasi-Monte Carlo, partial differential equations, singular limits, uncertainty quantification
Related publications
Hauck, Moritz; Målqvist, Axel; Rupp, Andreas
Arbitrary order approximations at constant cost for Timoshenko beam network models Online Forthcoming
Forthcoming.
@online{HauckMR24,
title = {Arbitrary order approximations at constant cost for Timoshenko beam network models},
author = {Moritz Hauck and Axel Målqvist and Andreas Rupp},
url = {https://arxiv.org/abs/2407.14388},
year = {2024},
date = {2024-07-19},
abstract = {This paper considers the numerical solution of Timoshenko beam network models, comprised of Timoshenko beam equations on each edge of the network, which are coupled at the nodes of the network using rigid joint conditions. Through hybridization, we can equivalently reformulate the problem as a symmetric positive definite system of linear equations posed on the network nodes. This is possible since the nodes, where the beam equations are coupled, are zero-dimensional objects. To discretize the beam network model, we propose a hybridizable discontinuous Galerkin method that can achieve arbitrary orders of convergence under mesh refinement without increasing the size of the global system matrix. As a preconditioner for the typically very poorly conditioned global system matrix, we employ a two-level overlapping additive Schwarz method. We prove uniform convergence of the corresponding preconditioned conjugate gradient method under appropriate connectivity assumptions on the network. Numerical experiments support the theoretical findings of this work.},
keywords = {},
pubstate = {forthcoming},
tppubtype = {online}
}
This paper considers the numerical solution of Timoshenko beam network models, comprised of Timoshenko beam equations on each edge of the network, which are coupled at the nodes of the network using rigid joint conditions. Through hybridization, we can equivalently reformulate the problem as a symmetric positive definite system of linear equations posed on the network nodes. This is possible since the nodes, where the beam equations are coupled, are zero-dimensional objects. To discretize the beam network model, we propose a hybridizable discontinuous Galerkin method that can achieve arbitrary orders of convergence under mesh refinement without increasing the size of the global system matrix. As a preconditioner for the typically very poorly conditioned global system matrix, we employ a two-level overlapping additive Schwarz method. We prove uniform convergence of the corresponding preconditioned conjugate gradient method under appropriate connectivity assumptions on the network. Numerical experiments support the theoretical findings of this work.
Vedral, Joshua; Rupp, Andreas; Kuzmin, Dmitri
Strongly consistent low-dissipation WENO schemes for finite elements Online Forthcoming
Forthcoming, visited: 08.07.2024.
@online{VedralRK24,
title = {Strongly consistent low-dissipation WENO schemes for finite elements},
author = {Joshua Vedral and Andreas Rupp and Dmitri Kuzmin},
url = {https://arxiv.org/abs/2407.04646},
year = {2024},
date = {2024-07-08},
urldate = {2024-07-08},
abstract = {We propose a way to maintain strong consistency and facilitate error analysis in the context of dissipation-based WENO stabilization for continuous and discontinuous Galerkin discretizations of conservation laws. Following Kuzmin and Vedral (J. Comput. Phys. 487:112153, 2023) and Vedral (arXiv preprint arXiv:2309.12019), we use WENO shock detectors to determine appropriate amounts of low-order artificial viscosity. In contrast to existing WENO methods, our approach blends candidate polynomials using residual-based nonlinear weights. The shock-capturing terms of our stabilized Galerkin methods vanish if residuals do. This enables us to achieve improved accuracy compared to weakly consistent alternatives. As we show in the context of steady convection-diffusion-reaction (CDR) equations, nonlinear local projection stabilization terms can be included in a way that preserves the coercivity of local bilinear forms. For the corresponding Galerkin-WENO discretization of a CDR problem, we rigorously derive a priori error estimates. Additionally, we demonstrate the stability and accuracy of the proposed method through one- and two-dimensional numerical experiments for hyperbolic conservation laws and systems thereof. The numerical results for representative test problems are superior to those obtained with traditional WENO schemes, particularly in scenarios involving shocks and steep gradients.},
keywords = {},
pubstate = {forthcoming},
tppubtype = {online}
}
We propose a way to maintain strong consistency and facilitate error analysis in the context of dissipation-based WENO stabilization for continuous and discontinuous Galerkin discretizations of conservation laws. Following Kuzmin and Vedral (J. Comput. Phys. 487:112153, 2023) and Vedral (arXiv preprint arXiv:2309.12019), we use WENO shock detectors to determine appropriate amounts of low-order artificial viscosity. In contrast to existing WENO methods, our approach blends candidate polynomials using residual-based nonlinear weights. The shock-capturing terms of our stabilized Galerkin methods vanish if residuals do. This enables us to achieve improved accuracy compared to weakly consistent alternatives. As we show in the context of steady convection-diffusion-reaction (CDR) equations, nonlinear local projection stabilization terms can be included in a way that preserves the coercivity of local bilinear forms. For the corresponding Galerkin-WENO discretization of a CDR problem, we rigorously derive a priori error estimates. Additionally, we demonstrate the stability and accuracy of the proposed method through one- and two-dimensional numerical experiments for hyperbolic conservation laws and systems thereof. The numerical results for representative test problems are superior to those obtained with traditional WENO schemes, particularly in scenarios involving shocks and steep gradients.
Cheng, Hanz Martin; Helin, Tapio; Manninen, Ville-Petteri; Holopainen, Timo; Jokinen, Juha; Sorvari, Samu; Rupp, Andreas
Recovery of transversely-isotropic elastic material parameters in induction motor rotors Online Forthcoming
Forthcoming, visited: 10.05.2024.
@online{ChengHMHJSR24,
title = {Recovery of transversely-isotropic elastic material parameters in induction motor rotors},
author = {Hanz Martin Cheng and Tapio Helin and Ville-Petteri Manninen and Timo Holopainen and Juha Jokinen and Samu Sorvari and Andreas Rupp},
url = {https://arxiv.org/abs/2405.06388},
year = {2024},
date = {2024-05-10},
urldate = {2024-05-10},
abstract = {We propose numerical algorithms for recovering parameters in eigenvalue problems for linear elasticity of transversely isotropic materials. Specifically, the algorithms are used to recover the elastic constants of a rotor core. Numerical tests show that in the noiseless setup, two pairs of bending modes are sufficient for recovering one to four parameters accurately. To recover all five parameters that govern the elastic properties of electric engines accurately, we require three pairs of bending modes and one torsional mode. Moreover, we study the stability of the inversion method against multiplicative noise; for tests in which the data contained multiplicative noise of at most 1%, we find that all parameters can be recovered with an error less than 10%. },
keywords = {},
pubstate = {forthcoming},
tppubtype = {online}
}
We propose numerical algorithms for recovering parameters in eigenvalue problems for linear elasticity of transversely isotropic materials. Specifically, the algorithms are used to recover the elastic constants of a rotor core. Numerical tests show that in the noiseless setup, two pairs of bending modes are sufficient for recovering one to four parameters accurately. To recover all five parameters that govern the elastic properties of electric engines accurately, we require three pairs of bending modes and one torsional mode. Moreover, we study the stability of the inversion method against multiplicative noise; for tests in which the data contained multiplicative noise of at most 1%, we find that all parameters can be recovered with an error less than 10%.
Di Pietro, Daniele Antonio; Dong, Zhaonan; Kanschat, Guido; Matalon, Pierre; Rupp, Andreas
Homogeneous multigrid for hybrid discretizations: application to HHO methods Online Forthcoming
Forthcoming, visited: 25.03.2024.
@online{DiPietroDKMR24,
title = {Homogeneous multigrid for hybrid discretizations: application to HHO methods},
author = {Di Pietro, Daniele Antonio and Zhaonan Dong and Guido Kanschat and Pierre Matalon and Andreas Rupp},
url = {https://arxiv.org/abs/2403.15858
https://inria.hal.science/hal-04518103},
year = {2024},
date = {2024-03-25},
urldate = {2024-03-25},
abstract = {We prove the uniform convergence of the geometric multigrid V-cycle for hybrid high-order (HHO) and other discontinuous skeletal methods. Our results generalize previously established results for HDG methods, and our multigrid method uses standard smoothers and local solvers that are bounded, convergent, and consistent. We use a weak version of elliptic regularity in our proofs. Numerical experiments confirm our theoretical results.},
keywords = {},
pubstate = {forthcoming},
tppubtype = {online}
}
We prove the uniform convergence of the geometric multigrid V-cycle for hybrid high-order (HHO) and other discontinuous skeletal methods. Our results generalize previously established results for HDG methods, and our multigrid method uses standard smoothers and local solvers that are bounded, convergent, and consistent. We use a weak version of elliptic regularity in our proofs. Numerical experiments confirm our theoretical results.
Lu, Peipei; Wang, Wei; Kanschat, Guido; Rupp, Andreas
Homogeneous multigrid for HDG applied to the Stokes equation Journal Article Forthcoming
In: IMA Journal of Numerical Analysis, Forthcoming, ISBN: 0272-4979.
@article{LuWKR23,
title = {Homogeneous multigrid for HDG applied to the Stokes equation},
author = {Peipei Lu and Wei Wang and Guido Kanschat and Andreas Rupp},
url = {https://academic.oup.com/imajna/advance-article-pdf/doi/10.1093/imanum/drad079/52422702/drad079.pdf},
doi = {10.1093/imanum/drad079},
isbn = {0272-4979},
year = {2023},
date = {2023-10-23},
urldate = {2023-10-23},
journal = {IMA Journal of Numerical Analysis},
abstract = {We propose a multigrid method to solve the linear system of equations arising from a hybrid discontinuous Galerkin (in particular, a single face hybridizable, a hybrid Raviart–Thomas, or a hybrid Brezzi–Douglas–Marini) discretization of a Stokes problem. Our analysis is centered around the augmented Lagrangian approach and we prove uniform convergence in this setting. Beyond this, we establish relations, which resemble those in Cockburn and Gopalakrishnan (2008, Error analysis of variable degree mixed methods for elliptic problems via hybridization. Math. Comput., 74, 1653–1677) for elliptic problems, between the approximates that are obtained by the single-face hybridizable, hybrid Raviart–Thomas and hybrid Brezzi–Douglas–Marini methods. Numerical experiments underline our analytical findings.},
keywords = {},
pubstate = {forthcoming},
tppubtype = {article}
}
We propose a multigrid method to solve the linear system of equations arising from a hybrid discontinuous Galerkin (in particular, a single face hybridizable, a hybrid Raviart–Thomas, or a hybrid Brezzi–Douglas–Marini) discretization of a Stokes problem. Our analysis is centered around the augmented Lagrangian approach and we prove uniform convergence in this setting. Beyond this, we establish relations, which resemble those in Cockburn and Gopalakrishnan (2008, Error analysis of variable degree mixed methods for elliptic problems via hybridization. Math. Comput., 74, 1653–1677) for elliptic problems, between the approximates that are obtained by the single-face hybridizable, hybrid Raviart–Thomas and hybrid Brezzi–Douglas–Marini methods. Numerical experiments underline our analytical findings.
Altunay, Rabia; Vesterinen, Kalevi; Alander, Pasi; Immonen, Eero; Rupp, Andreas; Roininen, Lassi
Denture reinforcement via topology optimization Online Forthcoming
Forthcoming, visited: 01.09.2023.
@online{AltunayVAIRR23,
title = {Denture reinforcement via topology optimization},
author = {Rabia Altunay and Kalevi Vesterinen and Pasi Alander and Eero Immonen and Andreas Rupp and Lassi Roininen},
url = {https://arxiv.org/abs/2309.00396},
year = {2023},
date = {2023-09-01},
urldate = {2023-09-01},
abstract = {We present a computational design method that optimizes the reinforcement of dental prostheses and increases the durability and fracture resistance of dentures. Our approach optimally places reinforcement, which could be implemented by modern multi-material, three-dimensional printers. The study focuses on reducing deformation by identifying regions within the structure that require reinforcement (E-glass material). Our method is applied to a three-dimensional removable lower jaw dental prosthesis and aims to improve the living quality of denture patients and pretend fracture of dental reinforcement in clinical studies. To do this, we compare the deformation results of a non-reinforced denture and a reinforced denture that has two materials. The results indicate the maximum deformation is lower and node-based displacement distribution demonstrates that the average displacement distribution is much better in the reinforced denture.},
keywords = {},
pubstate = {forthcoming},
tppubtype = {online}
}
We present a computational design method that optimizes the reinforcement of dental prostheses and increases the durability and fracture resistance of dentures. Our approach optimally places reinforcement, which could be implemented by modern multi-material, three-dimensional printers. The study focuses on reducing deformation by identifying regions within the structure that require reinforcement (E-glass material). Our method is applied to a three-dimensional removable lower jaw dental prosthesis and aims to improve the living quality of denture patients and pretend fracture of dental reinforcement in clinical studies. To do this, we compare the deformation results of a non-reinforced denture and a reinforced denture that has two materials. The results indicate the maximum deformation is lower and node-based displacement distribution demonstrates that the average displacement distribution is much better in the reinforced denture.
Lu, Peipei; Maier, Roland; Rupp, Andreas
A localized orthogonal decomposition strategy for hybrid discontinuous Galerkin methods Online Forthcoming
Forthcoming, visited: 28.07.2023.
@online{LuMR23,
title = {A localized orthogonal decomposition strategy for hybrid discontinuous Galerkin methods},
author = {Peipei Lu and Roland Maier and Andreas Rupp},
url = {https://arxiv.org/abs/2307.14961},
year = {2023},
date = {2023-07-28},
urldate = {2023-07-28},
abstract = {We formulate and analyze a multiscale method for an elliptic problem with an oscillatory coefficient based on a skeletal (hybrid) formulation. More precisely, we employ hybrid discontinuous Galerkin approaches and combine them with the localized orthogonal decomposition methodology to obtain a coarse-scale skeletal method that effectively includes fine-scale information. This work is a first step to reliably merge hybrid skeletal formulations and localized orthogonal decomposition and unite the advantages of both strategies. Numerical experiments are presented to illustrate the theoretical findings. },
keywords = {},
pubstate = {forthcoming},
tppubtype = {online}
}
We formulate and analyze a multiscale method for an elliptic problem with an oscillatory coefficient based on a skeletal (hybrid) formulation. More precisely, we employ hybrid discontinuous Galerkin approaches and combine them with the localized orthogonal decomposition methodology to obtain a coarse-scale skeletal method that effectively includes fine-scale information. This work is a first step to reliably merge hybrid skeletal formulations and localized orthogonal decomposition and unite the advantages of both strategies. Numerical experiments are presented to illustrate the theoretical findings.
Kazarnikov, Alexey; Ray, Nadja; Haario, Heikki; Lappalainen, Joona; Rupp, Andreas
Parameter estimation for cellular automata Online Forthcoming
Forthcoming, visited: 01.02.2023.
@online{KazarnikovRHLR23,
title = {Parameter estimation for cellular automata},
author = {Alexey Kazarnikov and Nadja Ray and Heikki Haario and Joona Lappalainen and Andreas Rupp},
url = {https://arxiv.org/abs/2301.13320},
year = {2023},
date = {2023-02-01},
urldate = {2023-02-01},
abstract = {Self organizing complex systems can be modeled using cellular automaton models. However, the parametrization of these models is crucial and significantly determines the resulting structural pattern. In this research, we introduce and successfully apply a sound statistical method to estimate these parameters. The method is based on constructing Gaussian likelihoods using characteristics of the structures such as the mean particle size. We show that our approach is robust with respect to the method parameters, domain size of patterns, or CA iterations. },
keywords = {},
pubstate = {forthcoming},
tppubtype = {online}
}
Self organizing complex systems can be modeled using cellular automaton models. However, the parametrization of these models is crucial and significantly determines the resulting structural pattern. In this research, we introduce and successfully apply a sound statistical method to estimate these parameters. The method is based on constructing Gaussian likelihoods using characteristics of the structures such as the mean particle size. We show that our approach is robust with respect to the method parameters, domain size of patterns, or CA iterations.
Kaarnioja, Vesa; Rupp, Andreas
Quasi-Monte Carlo and discontinuous Galerkin Online Forthcoming
Forthcoming, visited: 15.07.2022.
@online{KaarniojaR22,
title = {Quasi-Monte Carlo and discontinuous Galerkin},
author = {Vesa Kaarnioja and Andreas Rupp},
url = {https://arxiv.org/abs/2207.07698},
year = {2022},
date = {2022-07-15},
urldate = {2022-07-15},
abstract = {In this study, we design and develop Quasi-Monte Carlo (QMC) cubatures for non-conforming discontinuous Galerkin (DG) approximations of elliptic partial differential equations (PDEs) with random coefficient. That is, we consider the affine and uniform, and the lognormal models for the input random field, and investigate the QMC cubatures to compute the response statistics (expectation and variance) of the discretized PDE. In particular, we prove that the resulting QMC convergence rate for DG approximations behaves in the same way as if continuous finite elements were chosen. Numerical results underline our analytical findings. Moreover, we present a novel analysis for the parametric regularity in the lognormal setting.},
keywords = {},
pubstate = {forthcoming},
tppubtype = {online}
}
In this study, we design and develop Quasi-Monte Carlo (QMC) cubatures for non-conforming discontinuous Galerkin (DG) approximations of elliptic partial differential equations (PDEs) with random coefficient. That is, we consider the affine and uniform, and the lognormal models for the input random field, and investigate the QMC cubatures to compute the response statistics (expectation and variance) of the discretized PDE. In particular, we prove that the resulting QMC convergence rate for DG approximations behaves in the same way as if continuous finite elements were chosen. Numerical results underline our analytical findings. Moreover, we present a novel analysis for the parametric regularity in the lognormal setting.