Research Projects
DREAM: Doctoral education pilot for mathematics of sensing, imaging and modelling

Duration: 08/2024 – 12/2027
Funding scheme: Call for Doctoral Education Pilot Projects in Finnish Flagship Areas
Funder: Finnish Ministry of Education and Culture
Budget: 25,500,000 €
My role: Principal investigator
Main responsible: Tanja Tarvainen
Parent project: FAME: Flagship of advanced mathematics for sensing, imaging and modelling
Imaging and sensing challenges are pervasive across various sectors of society and industry. Cost-effective advancements in imaging, diagnostics, and therapeutics are imperative for improving healthcare accessibility and quality. Similarly, the materials and process industry needs more non-destructive testing methods crucial for safety, energy efficiency, and sustainability. Environmental endeavors like biodiversity monitoring and climate change prediction also encounter similar hurdles.
These challenges often fall into the category of ill-posed inverse problems, necessitating specialized techniques rooted in inverse mathematics. DREAM draws upon the methodological expertise of the Centre of Excellence in Inverse Modeling and Imaging and the Flagship of Advanced Mathematics for Sensing, Imaging, and Modeling (FAME). FAME, a leading international competence center, aims to provide solutions for imaging and sensing problems, contributing to the well-being of Finnish society and the global economy.
DREAM focuses on training experts to develop methodologies essential for diverse industrial and societal applications. The acquired expertise is versatile and applicable across scientific disciplines, industries, and society. Collaborations with stakeholders, particularly in healthcare and environmental sustainability, offer extensive opportunities for doctoral candidates. Consequently, DREAM is poised to facilitate knowledge transfer, foster competence-based growth, and address crucial societal needs.
Localized orthogonal decomposition for high-order, hybrid finite elements
Duration: 03/2024 – 02/2026
Funding scheme: Funding for Mobility Cooperation with Germany
Funder: Research Council of Finland
Budget: 18,000 €
My role: Finnish principal investigator
Sibling project: High-order hybrid multiscale methods for rough heterogeneous structures
German principal investigator: Roland Maier
Many natural processes involve multiple scales, such as fluid flow in porous materials or wave propagation through layered soil. These systems have microscopic scales, describing material properties and textures, and macroscopic scales, where observable phenomena occur. The same principle applies in manufacturing, for example, when producing fiber-reinforced composites that alter material behavior.
Mathematically, partial differential equations with coefficients varying on a microscopic scale describe such processes. While typically only practical macroscopic information is of interest, discarding microscopic features in numerical simulations often fails to provide the desired results. However, fully resolving microscopic coefficients is computationally prohibitive.
Numerical homogenization methods offer a possible solution to this problem. This project aims to improve their performance by combining state-of-the-art hybrid finite elements with high-order multiscale approaches.
High-order hybrid multiscale methods for rough heterogeneous structures
Duration: 01/2024 – 12/2025
Funding scheme: Program for Project-Related Personal Exchange with Finland
Funder: German Academic Exchange Service
Budget: 15,907 €
My role: Finnish principal investigator
Sibling project: Localized orthogonal decomposition for high-order, hybrid finite elements
German principal investigator: Roland Maier
Multiscale problems occur in many relevant applications and involve multiple scales requiring an appropriate treatment to avoid costly and unfeasible computations. Multiscale methods tackle this problem and construct problem-adapted approximation spaces that suitably include fine-scale information while operating on a coarse scale of interest. Many multiscale methods that fall under this category have been developed recently. Focusing on minimal assumptions on the microstructure in an elliptic setting, particularly generalized (multiscale) finite element methods, the localized orthogonal decomposition method (LOD), and gamblets may be mentioned.
Virtually all methods above are conforming, but researchers have turned to hybrid finite element methods (HFEMs) to aim for more local shape functions and a smaller (compared to non-conforming methods), sparse system of linear equations, which is symmetric positive definite for many dual finite element approximations. Many HFEMs exhibit several further advantageous properties, such as the possibility of boosting the order of convergence via postprocessing. The beneficial properties of HFEMs have also led to their use in the multiscale context. However, these multiscale methods require additional (piecewise) regularity assumptions concerning (at least) the coarse scale of interest.
The PIs have constructed the first combination of the LOD and HFE methodologies mentioned above and have proven their convergence properties under minimal regularity assumptions. However, their result is only a first step towards the reliable exploitation of the advantages of both techniques. This project aims to construct a combination of the LOD and HFE methods superior to the available techniques, building upon the PIs’ previous work.
FAME: Flagship of advanced mathematics for sensing, imaging and modelling

Duration: 01/2024 – 04/2028
Funding scheme: Flagship Programme call 4
Funder: Research Council of Finland
Budget: 7,999,994 €
My role: Principal investigator
Main responsible: Tanja Tarvainen (director), Nuutti Hyvönen (vice director), Samuli Siltanen (deputy vice director)
Links: Flagship description by the Research Council of Finland, official FAME website
Child project: DREAM: Doctoral education pilot for mathematics of sensing, imaging and modelling
The FAME Flagship brings the power of mathematics and physics to the service of society. Its academic team has a wealth of experience in developing inversion methods for medical imaging, industrial process monitoring, testing structures for defects without breaking them, and interpreting satellite data. The generality of mathematics allows transferring the techniques from one application area to another. Industrial partners of FAME, working with healthcare, clean industry, and sustainable environment, will co-create novel solutions for their technologies with the FAME team. Furthermore, their goals will give rise to new scientific questions. Science communication is a focus area of FAME. Researchers train to discuss science with general audiences and young people, leading to a broader appreciation for science and mathematics. FAME will support economic progress in Finland through intense interaction with industry, training of experts, and boosting innovations and start-up companies.
Uncertainty quantification for PDEs on hypergraphs
Duration: 09/2023 – 08/2027
Funding scheme: Academy Research Fellow
Funder: Academy of Finland
Budget: 667,532 € + 286,086 €
My role: Principal investigator
Link: https://research.fi/en/results/funding/78093
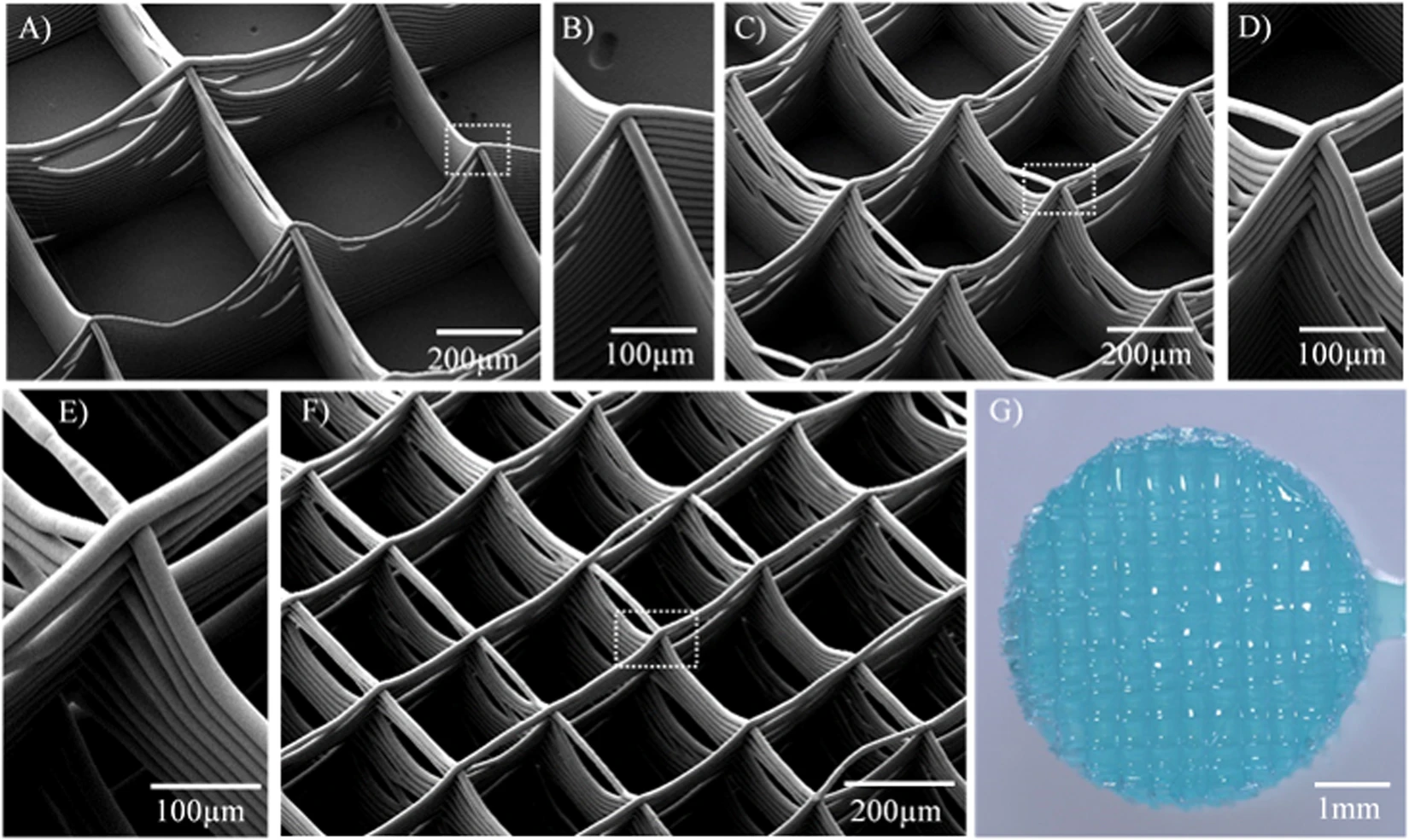
3D printing is becoming ubiquitous in engineering and science. One of the main reasons for such success is its ability to create small structures not producible in any other known way. Typical examples include lightweight but strong materials (resembling, e.g., honeycombs) and artificial tissue. Such materials need specific properties, while production is subject to uncertainties in the printing process. This project grows out of the need for mathematical algorithms to find optimal structures that retain their outstanding properties even in the presence of small errors. Robustness is vital for lightweight materials used to build lighter cars, planes, and rockets that save fuel. Similarly, 3D-printed artificial tissue has to mimic the real human tissue of fire victims to a high degree.
Compared to the most efficient existing approaches, the methodology of this project reduces the cost of an optimization-based product design cycle by orders of magnitude.
The picture shows micro-fiber scaffolds that are one artificial tissue component. It is taken from Mechanical behavior of a soft hydrogel reinforced with three-dimensional printed microfibre scaffolds by Miguel Castilho et al., licensed under CC BY 4.0.
3D-Cure: 3D printing for personalized medicine and customized drug delivery
Duration: 05/2023 – 04/2025
Funder: Business Finland
My role: Project manager
Main responsible: Eero Immonen
Unfortunately, the details of this project are confidential.
Mathematical models and numerical methods for water management in soils
Duration: 02/2022 – 02/2024
Funding scheme: Funding for Mobility Cooperation with Germany
Funder: Academy of Finland
Budget: 18,000 €
My role: Finnish principal investigator
Sibling project: Discontinuous Galerkin methods and parameter estimation for microstructure models in porous media
German principal investigator: Nadja Ray

Nowadays, soils should meet more and more requirements. For example, they should be stable to build houses on them safely. In flood-prone areas, they should be able to absorb a lot of water, and in arid regions, they should hold water as well as possible. Soils also play an essential role in the greenhouse effect, as they (and not just the plants growing on their surface) can sequester enormous amounts of carbon and release it if not treated properly.
However, if we want to treat soils to meet all or at least some of these requirements, we need to understand the basic building blocks (i.e., microaggregates; see the picture) of soils and how their development affects soil properties. To this end, we develop two models and numerical methods to simulate them. First, we describe the formation of soil microaggregates, and second, we consider the flow of fluids through consolidated soil. Our goal is to apply the results to support water management.
The image is taken from Microaggregates in soils by Kai Uwe Totsche et al., licensed under CC BY 4.0.
Discontinuous Galerkin methods and parameter estimation for microstructure models in porous media
Duration: 01/2022 – 12/2023
Funding scheme: Program for Project-Related Personal Exchange with Finland
Funder: German Academic Exchange Service
Budget: 15,892 €
My role: Finnish principal investigator
Sibling project: Mathematical models and numerical methods for water management in soils
German principal investigator: Nadja Ray
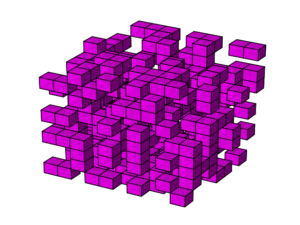
Due to their simplicity and flexibility, cellular automata (cellular automaton CA; an illustration is on the left) have been successfully used to describe many physical, sociological, technical, and economic processes, including modeling biological pattern formation such as tumor growth and cell adhesion processes, epidemics, traffic on roads, and biofilm growth. Together with other project participants, project leaders Nadja Ray and Andreas Rupp have developed and used CA models in joint preliminary work to describe the formation and turnover of microaggregates in soils. These microaggregates directly affect soils’ quality and efficient agricultural usability; thus, they are relevant for current socio-political issues. However, the validity of cellular automata strongly depends on the choice of appropriate parameters that balance the relevant and competing physical processes. In this project, we will develop methods to choose these parameters appropriately.
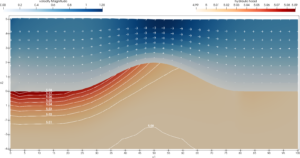
The coupling of free flow and subsurface flow is essential in numerous applications, such as infiltration of overland runoff into the ground during precipitation, contaminant dispersion into the subsurface, sedimentation processes, and interaction of oceans, lakes, rivers, or wetlands with aquifers. Based on previous results of the research leaders, this research topic will investigate the coupling of a free flow (Stokes equations) with the flow in the porous medium (Darcy equation), which is relevant in the context of energy production.